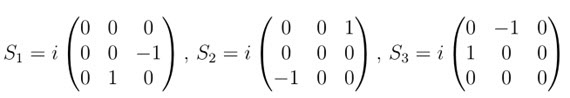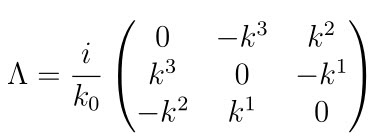Photon – amount of light. they say? But what is quantum? And what is light? Photon – a quantity of energy. But what is this “energy”? Photon – has a rotation. But what is all this spindle? Light – can be polarized. But what is this polarity?
It’s a little better with polarity. We can describe it mathematically – algebraically. Simple algebra. High school students can learn it easily. It’s just that they don’t know it. There is collusion in secondary schools. A high school student who masters algebra can become smarter than a teacher. This is why algebra is not taught in high schools. They know the binomial, but algebra does not. And algebra is matrices. Why don’t they teach mothers in schools? Why don’t they know the Quartet? This knowledge seems dangerous. It takes from reality to mysticism, to imaginary beings. It turns students’ heads. They know about the Holy Spirit, but they do not know about the Quartet. They know about the Holy Trinity, but not about the Trinity of Quartet I, j, k. Isaac Newton, a Kabbalist Jew, wrote much about the Trinity. He questioned this belief. Because he did not know the Quartet. Even less, he did not know octons.
The photon has rotation. The electron has a spin of 1/2. A photon has spin 1. Or to be precise: it has rotation and dynamism. The world, life, consciousness, information – all this is due to convolution. In English: Helicity. spirochetes; Planets rotate, galaxies rotate. Whirlpools, vortices, vortices over vortices. This is the secret of existence. Let’s take a closer look at it.
Three-dimensional space. Painter renders it on a two-dimensional level. Drawing of Saint Cezanne. Victoria.
Polish versions here.
How do you render a 3D space on a 2D plane? How do you work with light, colors and geometric shapes? How do you work with light?
Here’s how. The wave function of a photon consists of three complex functions F(k) = (f1 (K), f2 (K), f3 (K)). The argument is a wave vector K With a physical dimension equal to the reciprocal of length. Complex 3×3 matrices operate on 3 complex vectors. Here are the three basic spinning-related matrices:
The imaginary squares i, j, k have the properties:
IJ=KAnd J= –K
k=AndAnd Kj = –And
in order to=y ik = –y
Where did it come from, among other things
[ ½i, ½j] = ½K
where the inverter [A,B]= AB-BA is a basic hated process. Matrix multiplication vs multiplication of numbers (real or complex) is non interchangeable. And this is the great mystery and power of algebra,
Arrays Σ fulfill the same switching relationships as (half) imaginary quaternions
[Σ1,Σ2]= Σ3[Σ2,Σ3]= Σ1
[Σ3,Σ1]= Σ2
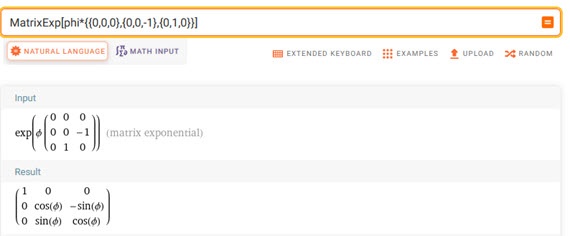
These are 3D rotation generators. We go to Wolfram Alpha and enter:
We get the rotation matrix with the angle phi about the x axis. Similarly, 2 is the generator of rotation around the y axis and similarly Σ3 – is the generator of rotation around the z axis. Cycles, swirls.
But we want to rotate the photon wave functions. Rotation related to zigzag. Skewness is a physical quantity. In quantum geometry, we associate hierarchical matrices with physical quantities. Arrays are anti-Hermitian. What does a physicist do? You multiply Σ by imaginary i, and you get hierarchical matrices:
He doesn’t have to think at all. He reached the stairs and pulled out the first imagination, without the complex numbers there would be no quantum mechanics. Mysterious Omnipotent 1. Base Minus 1. The greatest invention of all time. Euler’s formula shown below is considered one of the most beautiful mathematical formulas of all time; Euler – Mozart Mathematics, so they say:
So we have matrices S – Hermitian skew factors. From them we construct the factor of the skew matrix Λ:
Λ = (k1 S1 + k2 S2 + k3 S3) / k0
where k0 = √ (k12+ k22+ k32)
The factor Λ is Hermitian. It has eigenvalues of 0, -1, + 1. The last two are around the photon. The photon has two degrees of freedom. Just like Cezanne’s painting. We have to learn how to project from 3D space on a 2D photon canvas.
matrix Λ2 It is hierarchical and has eigenvalues of 0 and +1. It is a two-dimensional plane projection factor for photon states. It is better to introduce two factors to project on subspaces with a given skew
P1 = (Λ2+ Λ) / 2
P2 = (Λ2-Λ) / 2
Now P1 + P2 =2.
P1 projects into the photon states with +1 torsion, P2 projects into the photon states with torsion -1. Here is their public form
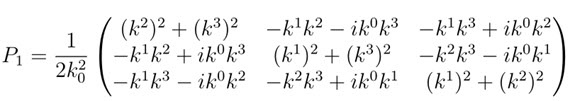
s.We also need the time inversion factor Θ:
(ΘF) (K) =F(-K) *
And now we can go back to the Riemann-Silberstein vector from previous noteI drove my car behind the Pyazinicki family by mistake. I suspected that they wanted to comb the uncollectible sphere. It was actually so. However, the Riemann-Silberstein vector can be inserted without combing the ball. Only yesterday I understood it. The formula is simple.
We take the photon wave function f (K, R). It may depend on time. And from it we build the Riemann-Silberstein vector:
F.(Kt) = P1 F(Kt) + P2 (ΘF) (KR)
If we want, we can compute the inverse Fourier transform F.(xR). The real part is the electric field, the imaginary part is the magnetic field. accounts and that’s it.
And there are no peculiarities associated with combing the non-combinable field. Because the matrices P1 and P2 do not contain any singularity. There is of course affinity for K= 0 but There are no photons without momentum.

Echo Richards embodies a personality that is a delightful contradiction: a humble musicaholic who never brags about her expansive knowledge of both classic and contemporary tunes. Infuriatingly modest, one would never know from a mere conversation how deeply entrenched she is in the world of music. This passion seamlessly translates into her problem-solving skills, with Echo often drawing inspiration from melodies and rhythms. A voracious reader, she dives deep into literature, using stories to influence her own hardcore writing. Her spirited advocacy for alcohol isn’t about mere indulgence, but about celebrating life’s poignant moments.